Finding General Term Of Sequence

Arithmetic Progression

An arithmetics progression (AP) is a sequence where the differences betwixt every ii consecutive terms are the same. In this blazon of progression, there is a possibility to derive a formula for the n^th term of the AP. For example, the sequence 2, half-dozen, x, 14, … is an arithmetic progression (AP) because information technology follows a blueprint where each number is obtained by adding 4 to the previous term. In this sequence, n^th term = 4n-ii. The terms of the sequence tin can be obtained by substituting n=one,2,iii,... in the n^thursday term. i.e.,

When n = i, offset term = 4n-2 = four(ane)-2 = 4-2=ii
When due north = 2, second term = 4n-two = iv(2)-2 = eight-ii=vi
When n = iii, thirs term = 4n-2 = four(iii)-2 = 12-2=10

In this article, we volition explore the concept of arithmetic progression, the formula to find its n^th term, common difference, and the sum of due north terms of an AP. We will solve various examples based on arithmetic progression formula for a improve understanding of the concept.

1.	What is Arithmetic Progression?
2.	Arithmetic Progression Formula
iii.	Mutual Terms Used in Arithmetics Progression
4.	Nth Term of Arithmetic Progression
5.	Sum of AP
six.	Differences Betwixt AP and GP
vii.	FAQs on Arithmetic Progression

What is Arithmetic Progression?

We can ascertain an arithmetic progression (AP) in two ways:

An arithmetic progression is a sequence where the differences betwixt every two sequent terms are the same.
An arithmetic progression is a sequence where each term, except the first term, is obtained by adding a fixed number to its previous term.

For example, 1, 5, 9, 13, 17, 21, 25, 29, 33, ... has

a = ane (the get-go term)
d = 4 (the "common departure" between terms)

In general an arithmetics sequence can be written like: {a, a+d, a+second, a+3d, ... }.

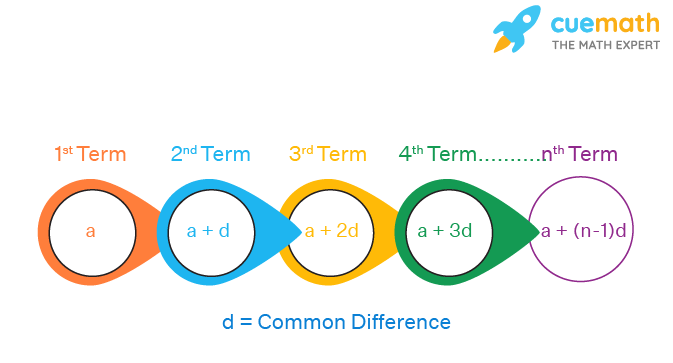
Arithmetic Progression

Using the above example we get: {a, a+d, a+2d, a+3d, ... } = {1, 1+4, one+2×4, 1+3×4, ... } = {1, five, 9, 13, ... }

Arithmetic Progression Definition

Arithmetic progression is defined as the sequence of numbers in algebra such that the divergence between every consecutive term is the aforementioned. It can be obtained by adding a fixed number to each previous term.

Arithmetics Progression Formula

For the showtime term 'a' of an AP and common difference 'd', given below is a list of arithmetic progression formulas that are usually used to solve various problems related to AP:

Common deviation of an AP: d = a_two - a₁= a_three - a₂ = a₄ - a_iii = ... = a_northward - a_{due north-1}
n^th term of an AP: a_north = a + (n - i)d
Sum of n terms of an AP: S_n= n/2(2a+(northward-1)d) = north/2(a + l), where l is the terminal term of the arithmetics progression.

AP Formula

The image beneath shows the formulas related to arithmetic progression:

Arithmetic Progression Formula

Mutual Terms Used in Arithmetics Progression

From now on, we will abridge arithmetics progression as AP. Here are some more than AP examples:

six, 13, 20, 27, 34, . . . .
91, 81, 71, 61, 51, . . . .
π, 2π, 3π, 4π, 5π,…
-√iii, −ii√3, −3√3, −4√3, −five√3,…

An AP mostly is shown as follows: a₁, a₂, a₃, . . . It involves the following terminology.

Outset Term of Arithmetic Progression:

As the name suggests, the outset term of an AP is the start number of the progression. It is usually represented by a₁ (or) a. For example, in the sequence 6,13,20,27,34, . . . . the offset term is 6. i.e., a₁=6 (or) a=six.

Common Deviation of Arithmetics Progression:

Nosotros know that an AP is a sequence where each term, except the first term, is obtained by calculation a fixed number to its previous term. Here, the "fixed number" is called the "common deviation" and is denoted by 'd' i.eastward., if the offset term is a_ane, so: the 2nd term is a₁+d, the tertiary term is a₁+d+d = a_ane+2nd, and the 4th term is a₁+2d+d= a_one+3d and and then on. For case, in the sequence half dozen,thirteen,twenty,27,34,. . . , each term, except the start term, is obtained past addition of 7 to its previous term. Thus, the mutual difference is, d=vii. In general, the common difference is the difference between every two successive terms of an AP. Thus, the formula for calculating the mutual difference of an AP is: d = a_n - a_n-1

Nth Term of Arithmetic Progression

The general term (or) n^th term of an AP whose first term is 'a' and the mutual difference is 'd' is found past the formula a_n=a+(n-i)d. For case, to detect the general term (or) n^th term of the sequence six,xiii,20,27,34,. . . ., we substitute the first term, a₁=6, and the common difference, d=7 in the formula for the n^th term formula. So we get, a_n=a+(north-1)d = 6+(n-i)7 = half-dozen+7n-7 = 7n -ane. Thus, the general term (or) due north^th term of this sequence is: a_n = 7n-i. Just what is the use of finding the general term of an AP? Let us see.

AP Formula for General Term

Nosotros know that to find a term, we can add 'd' to its previous term. For example, if we have to find the vi^th term of half-dozen,13,20,27,34, . . ., we can simply add d=7 to the v^th term which is 34. 6^thursdayterm = v^th term + 7 = 34+7 = 41. Simply what if we have to observe the 102^ndterm? Isn't it difficult to calculate it manually? In this case, nosotros can only substitute n=102 (and also a=vi and d=7 in the formula of the n^th term of an AP). Then we get:

a_n = a+(n-1)d

a₁₀₂ = 6+(102-1)7

a₁₀₂ = 6+(101)7

a₁₀₂ = 713

Therefore, the 102^nd term of the given sequence vi,13,20,27,34,.... is 713. Thus, the general term (or) n^th term of an AP is referred to as the arithmetic sequence explicit formula and can be used to discover whatever term of the AP without finding its previous term.

The following tabular array shows some AP examples and the first term, the common divergence, and the general term in each example.

Arithmetic Progression	Showtime Term	Common Difference	Full general Term due north^th term
AP	a	d	a_n= a + (due north-1)d
91,81,71,61,51, . . .	91	-ten	-10n+101
π,2π,3π,4π,5π,…	π	π	πn
–√three, −2√iii, −3√three, −iv√3–,…	-√3	-√three	-√3 n

Sum of Arithmetic Progression

Consider an arithmetic progression (AP) whose beginning term is a_one (or) a and the mutual difference is d.

The sum of first due north terms of an arithmetic progression when the n^th term is NOT known is South_n= (n/2)[2a+(due north-1) d]
The sum of kickoff n terms of an arithmetic progression when the n^thursday term, a_n is known is S_n= due north/2[a₁+a_n]

Example: Mr. Kevin earns $400,000 per annum and his salary increases by $fifty,000 per annum. And then how much does he earn at the end of the commencement iii years?

Solution: The amount earned by Mr. Kevin for the first twelvemonth is, a = 4,00,000. The increment per annum is, d = 50,000. We have to calculate his earnings in the iii years. So northward=3.

Substituting these values in the AP sum formula,

S_n=n/ii[2a+(due north-one) d]

Due south_north= 3/two(2(400000)+(iii-1)(50000))

= iii/2 (800000+100000)

= 3/two (900000)

= 1350000

He earned $1,350,000 in 3 years.

We can get the same answer by general thinking also as follows: The almanac corporeality earned past Mr. Kevin in the first iii years is as follows. This could be calculated manually every bit due north is a smaller value. But the above formulas are useful when n is a larger value.

Derivation of AP Sum Formula

Arithmetics progression is a progression in which every term later the first is obtained by adding a abiding value, chosen the mutual difference (d). So, to observe the n^th term of an arithmetic progression, we know a_{due north} = a₁ + (due north – one)d. a₁is the showtime term, a_i + d is the 2d term, the 3rd term is a₁ + 2nd, and and then on. For finding the sum of the arithmetic serial, S_{due north}, nosotros start with the starting time term and successively add the common difference.

Southward_{due north} = a₁ + (a₁ + d) + (a₁ + 2d) + … + [a_one + (northward–1)d].

We tin also start with the north^th term and successively subtract the mutual difference, so,

Southward_northward = a_{due north} + (a_n – d) + (a_n – 2nd) + … + [a_n – (due north–1)d].

Thus the sum of the arithmetic sequence could be constitute in either of the ways. However, on adding those two equations together, we get

Due south_n = a₁ + (a₁ + d) + (a_one + second) + … + [a_ane + (n–ane)d]

S_{due north} = a_n + (a_n – d) + (a_n – 2d) + … + [a_n – (north–i)d]

_________________________________________

2S_n = (a_i + a_{due north}) + (a₁ + a_northward) + (a₁ + a_n) + … + [a₁ + a_n].

____________________________________________

Observe all the d terms are cancelled out. So,

2S_north = n (a₁ + a_n)

⇒ Due south_n = [northward(a_ane + a_n)]/two --- (1)

By substituting a_n = a_one + (n – 1)d into the last formula, nosotros have

S_n = n/ii [a_one + a₁ + (n – ane)d] ...Simplifying

S_{due north} = due north/2 [2a₁ + (due north – one)d] --- (2)

These two formulas (1) and (2) help us to find the sum of an arithmetic series chop-chop. We can see the above derivation in the figure beneath.

Sum of Arithmetic Progression

Differences Betwixt Arithmetic Progression and Geometric Progression

The following table explains the differences betwixt arithmetic and geometric progression:

Arithmetic progression	Geometric progression
Arithmetic progression is a series in which the new term is the difference between two consecutive terms such that they have a constant value.	Geometric progression is divers equally the series in which the new term is obtained past multiplying the two consecutive terms such that they have a constant gene.
The series is identified as an arithmetics progression with the help of a mutual divergence betwixt consecutive terms.	The series is identified as a geometric progression with the help of a mutual ratio between consecutive terms.
The consecutive terms vary linearly.	The consecutive terms vary exponentially.

Important Notes on Arithmetic Progression

An AP is a list of numbers in which each term is obtained past adding a stock-still number to the preceding number.
a is represented equally the first term, d is a common difference, a_n as the nth term, and due north every bit the number of terms.
In general, AP can be represented every bit a, a+d, a+2nd, a+3d,..
the n^th term of an AP can exist obtained every bit a_n= a + (n−1)d
The sum of an AP can be obtained equally either s_n=north/2[2a+(north−one)d]
The graph of an AP is a straight line with the slope as the common difference.
The mutual difference doesn't need to be positive always. For instance, in the sequence, 16,8,0,−8,−16,.... the common difference is negative (d = viii - 16 = 0 - eight = -8 - 0 = -16 - (-eight) =... = -8).

☛ Related Articles:

Sum of Arithmetics Sequence Reckoner
Arithmetic Sequence Calculator
Sequence Calculator

Arithmetic Progression Examples

Example 1: Find the general term of the arithmetic progression -3, -(1/2), two…

Solution:

The given sequence is -3, -(1/two),2…

Hither, the first term is a=-3, and the common deviation is, d = -(1/2) -(-3) = -(1/2)+three = 5/2

By AP formulas, the full general term of an AP is calculated by the formula:

a_north = a+(n-ane)d

a_n = -3 +(due north-one) 5/two

= -iii+ (5/2)n - 5/two

= 5n/ii - 11/2

Therefore, the general term of the given AP is:

Reply: a_n = 5n/2 - 11/2
Instance 2: Which term of the AP 3, 8, 13, 18,... is 78?

Solution:

The given sequence is iii,eight,13,18,...

Hither the commencement term is a=3, and the common difference is, d = 8-three= xiii-8=...=five

Let u.s. assume that the n^th term is,

a_n=78

Substitute all these values in the general term of an arithmetic progression:
a_n = a+(n-ane)d
78 = 3+(n-ane)five
78 = three+5n-5
78 = 5n -two
fourscore = 5n
16 = n

Answer: ∴ 78 is the 16^th term
Example 3: Find the sum of the first five terms of the arithmetic progression whose first term is iii and 5^thursday term is xi.

Solution: We have a₁ = a = 3 and a₅ = 11 and north = 5.

Using the AP formula for the sum of due north terms, we have

S_northward = (n/2) (a + a_n)

⇒ S₅ = (5/two) (3 + xi)

= (5/ii) × 14

= 35

Answer: The required sum of the outset 5 terms is 35.

go to slidego to slidego to slide

Breakdown tough concepts through unproblematic visuals.

Math will no longer be a tough subject, especially when you sympathize the concepts through visualizations.

Book a Free Trial Class

Arithmetics Progression Questions

go to slidego to slide

FAQs on Arithmetic Progression

What is the Meaning of Arithmetic Progression in Maths?

A sequence of numbers that has a common deviation betwixt any two consecutive numbers is called an arithmetic progression (A.P.). The example of A.P. is 3,6,ix,12,fifteen,18,21, … In uncomplicated words, we tin say that an arithmetic progression is a sequence of numbers where the difference betwixt each sequent term is the same.

What is AP formula?

Here are the AP formulas corresponding to the AP a, a + d, a + 2nd, a + 3d, . . . a + (n - one)d:

The formula to notice the nth term is: a_northward = a + (n – 1) × d
The formula to observe the sum of north terms is Due south_n = n/2[2a + (n − i) × d]

What is the Sum of N Terms of the Arithmetics Progression Formula?

The sum of first n terms of an arithmetic progression when the nth term is NOT known is S_n = n/ii[2a+(n-1)d]. The sum of the start n terms of an arithmetic progression when the nth term, a_n is known is Southward_north = north/ii[a₁+a_northward].

How to Find the Sum of Arithmetic Progression?

To find the sum of arithmetic progression, nosotros have to know the first term, the number of terms, and the common deviation between consecutive terms. And so the formula to discover the sum of an arithmetics progression is Southward_northward = n/2[2a + (north − 1) × d] where, a = outset term of arithmetics progression, north = number of terms in the arithmetics progression and d = common difference.

How to Observe Common Difference in Arithmetic Progression?

The common difference is the divergence betwixt each consecutive term in an arithmetics sequence. Therefore, you can say that the formula to find the mutual difference of an arithmetic sequence is: d = a_n - a_{n - i}, where a_n is the n^thursday term in the sequence, and a_{due north - 1} is the previous term in the sequence.

How to Discover First Term in Arithmetics Progression?

If we know 'd'(common difference) and any term (nth term) in the progression then we can find 'a'(first term). Example: two,4,6,8,…….

north^th term(of arithmetic progression) = a+ (n-1)d, a = first term of arithmetics progression, n = number of terms in the arithmetic progression and d = common difference.

Hither, a = two, d = 4 – two = 6 – iv = 2,

If fifth term is ten and d=2, then

a₅= a + 4d; 10 = a + 4(two); 10 = a + 8; a = 2.

What is the Divergence Betwixt Arithmetic Sequence and Arithmetics Progression?

Arithmetic Sequence/Arithmetics Series is the sum of the elements of Arithmetics Progression. Arithmetics Progression is whatever number of sequences inside any range that gives a common departure.

How to Notice Number of Terms in Arithmetic Progression?

The number of terms in an arithmetic progression can be merely plant by the division of the difference betwixt the last and first terms by the common difference, then add 1.

What are the Types of Progressions in Maths?

There are 3 types of progressions in Maths. They are:

Arithmetics Progression (AP)
Geometric Progression (GP)
Harmonic Progression (HP)

Where is Arithmetic Progression Used?

A real-life application of arithmetic progression is seen when you take a taxi. Once you lot ride a taxi y'all will be charged an initial charge per unit and then a per mile or per kilometer charge. This shows an arithmetic sequence that for every kilometer you will exist charged a certain fixed (abiding) rate plus the initial charge per unit.

What is Nth Term in Arithmetics Progression?

The 'n^th' term in an AP is a formula with 'due north' in it which enables you lot to notice any term of a sequence without having to go up from 1 term to the next. 'n' stands for the term number and so to find the l^th term nosotros would only substitute l in the formula a_northward = a+ (n-1)d in place of 'n'.

How to Observe d in Arithmetic Progression?

To observe d in an arithmetic progression, we take the difference betwixt any 2 consecutive terms of the AP. Information technology is always a term minus its previous term.

How do you Solve Arithmetic Progression Problems?

The post-obit formulas assist to solve arithmetic progression issues:

Common difference of an AP: d = a_northward - a_n-1.
n^th term of an AP: a_{due north} = a + (n - 1)d
Sum of due north terms of an AP: South_{due north}= n/2(2a+(n-1)d)

where, a = outset term of arithmetic progression, n = number of terms in the arithmetic progression, and d = common divergence.

What is Infinite Arithmetic Progression?

When the number of terms in an AP is infinite, we phone call it an space arithmetic progression. For case, 2, 4, 6, viii, 10, ... is an space AP; etc.